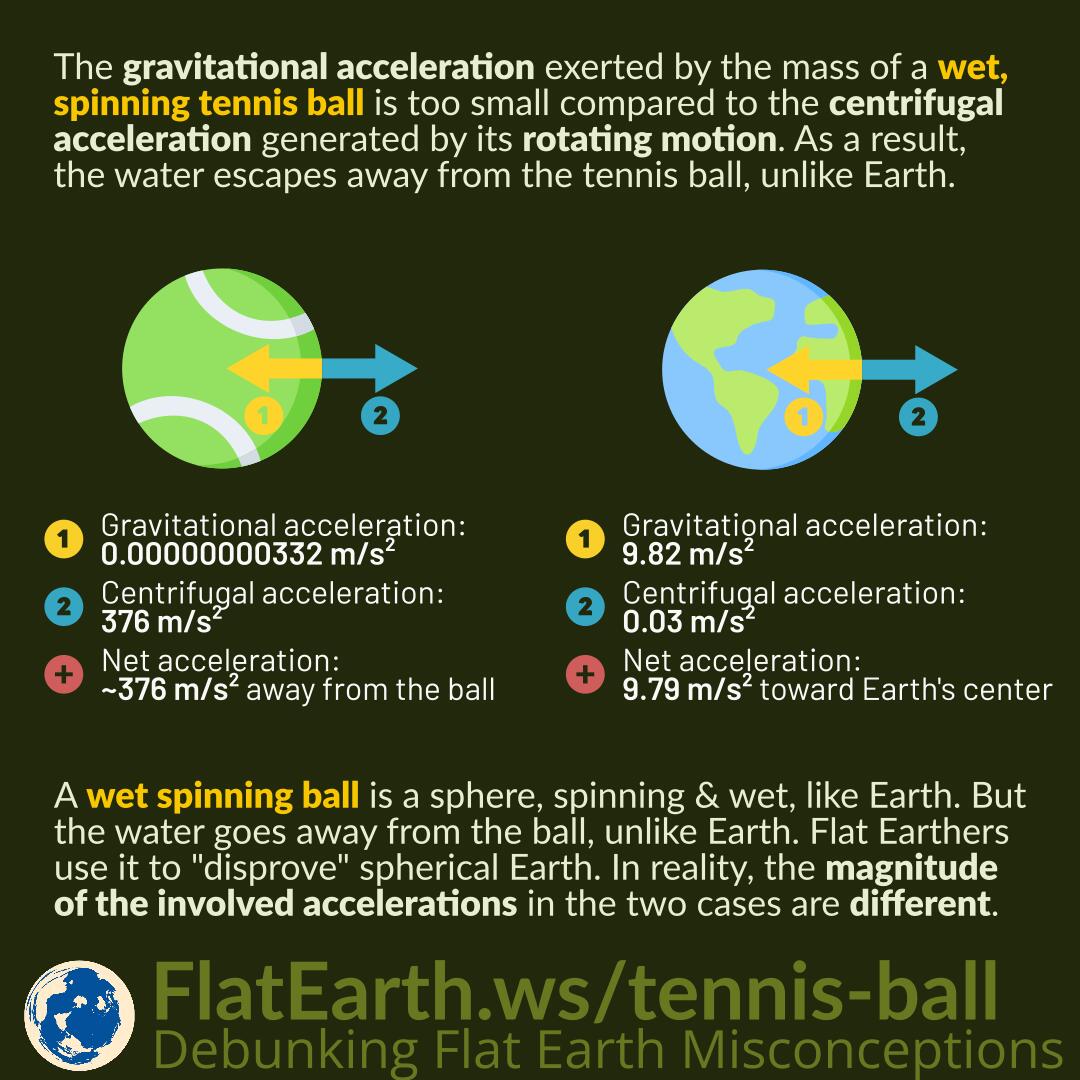
The gravitational acceleration exerted by the mass of a wet, spinning tennis ball is too small compared to the centrifugal acceleration generated by its rotating motion. As a result, the water escapes away from the tennis ball, unlike Earth.
A wet spinning ball is a sphere, spinning & wet, like Earth. But the water goes away from the ball, unlike Earth. Flat Earthers use it to “disprove” spherical Earth. In reality, the magnitude of the involved accelerations in the two cases are different.
Water remains on the surface of the Earth because Earth’s gravitational acceleration is greater than the centrifugal acceleration generated by its rotating motion. The Earth does not rotate nearly fast enough to produce the same magnitude of centrifugal acceleration caused by a spinning tennis ball.
Using Newton’s law of universal gravitation, we can find that the gravitational acceleration exerted by a tennis ball on an object on its surface is about 0.00000000332 m/s². On the other hand, its spinning motion generates a centrifugal acceleration of approximately 376 m/s², assuming the rotational rate of 1000 rpm. For comparison, Roger Federer’s backhand can create a spin of 5300 rpm. The net acceleration is about 376 m/s² away from the ball, causing water to fly away from the spinning ball.
Another consideration is that the spinning tennis ball “experiment” was performed on Earth and was affected by Earth’s gravity, several magnitudes greater than one from the tennis ball. Any water droplet on the tennis ball’s surface is influenced more by Earth’s gravity than the tennis ball.
Calculations
For the tennis ball:
- Diameter: 2.7 inches
- Mass: 58.5 gram
- Rotational velocity: assumed 1000 rpm (Roger Federer’s backhand can result in 5300 rpm spinning tennis ball).
- Centrifugal acceleration on the surface of a spinning tennis ball: a = ω²r = (((1000 / minutes) * (2 * π))^2) * (2.7 inch / 2) = 376.031928 m / s2
- Gravitational acceleration on the surface of a tennis ball: g = G M / r² = G * 58.5 gram / ( 2.7 inch / 2)^2 = 3.32056743 × 10-9 m / s2
For the Earth:
- Mass: 5.972 × 10^24 kg
- Radius: 6371 km
- Rotational velocity: 1/24 hours
- Gravitational acceleration o Earth’s surface: g = G M / r² = G * 5.972 × 10^24 kg / (6371 km)^2 = 9.81964974 m / s2
- Centrifugal acceleration o Earth’s surface: a = ω²r = (((1 / (24 hours)) * (2 * π))^2) * (6371 km) = 0.0336930136 m / s2
References
- Five things you may not know about Federer’s game – Wimbledon
- Tennis Ball – Wikipedia